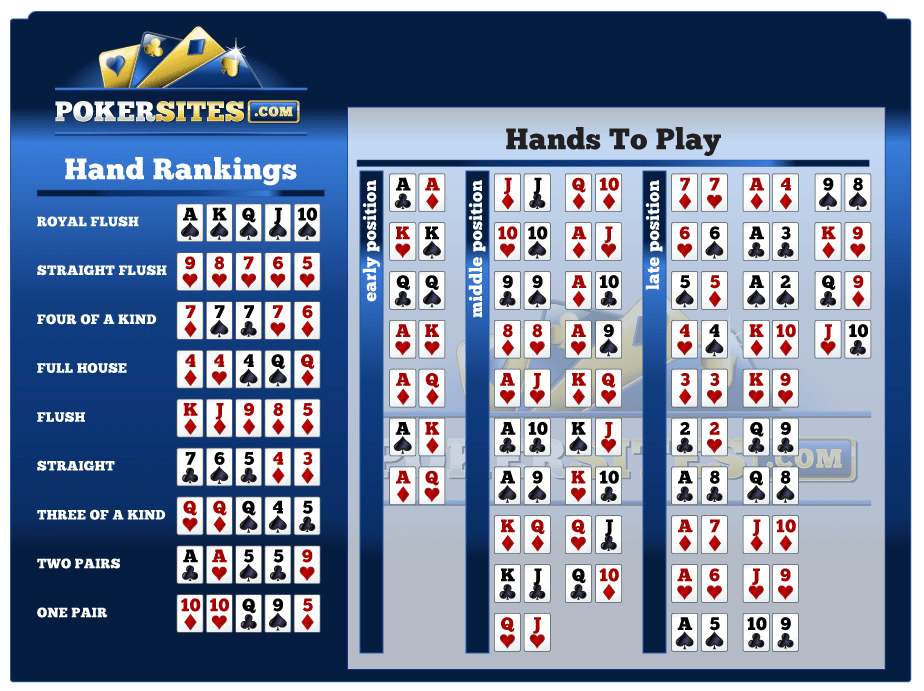
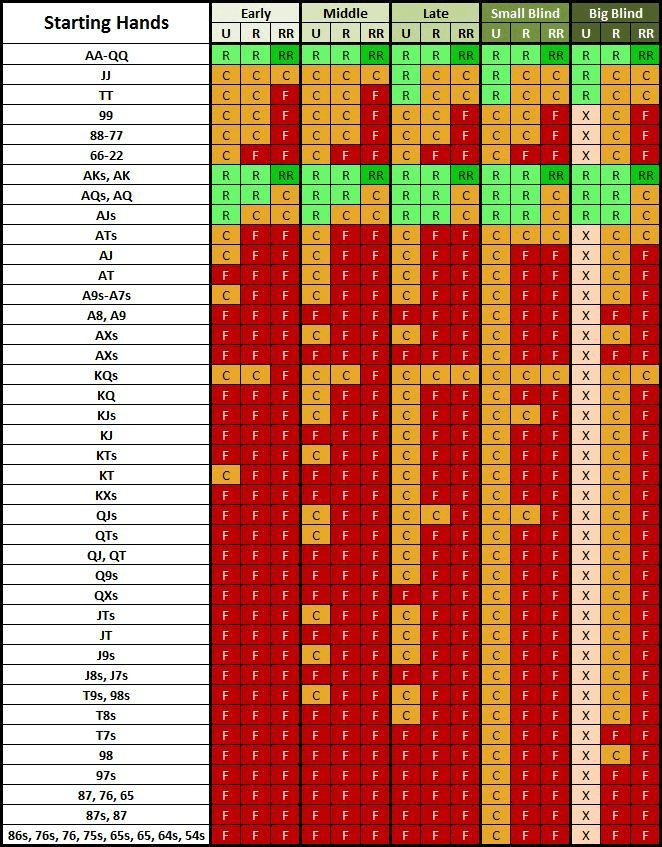
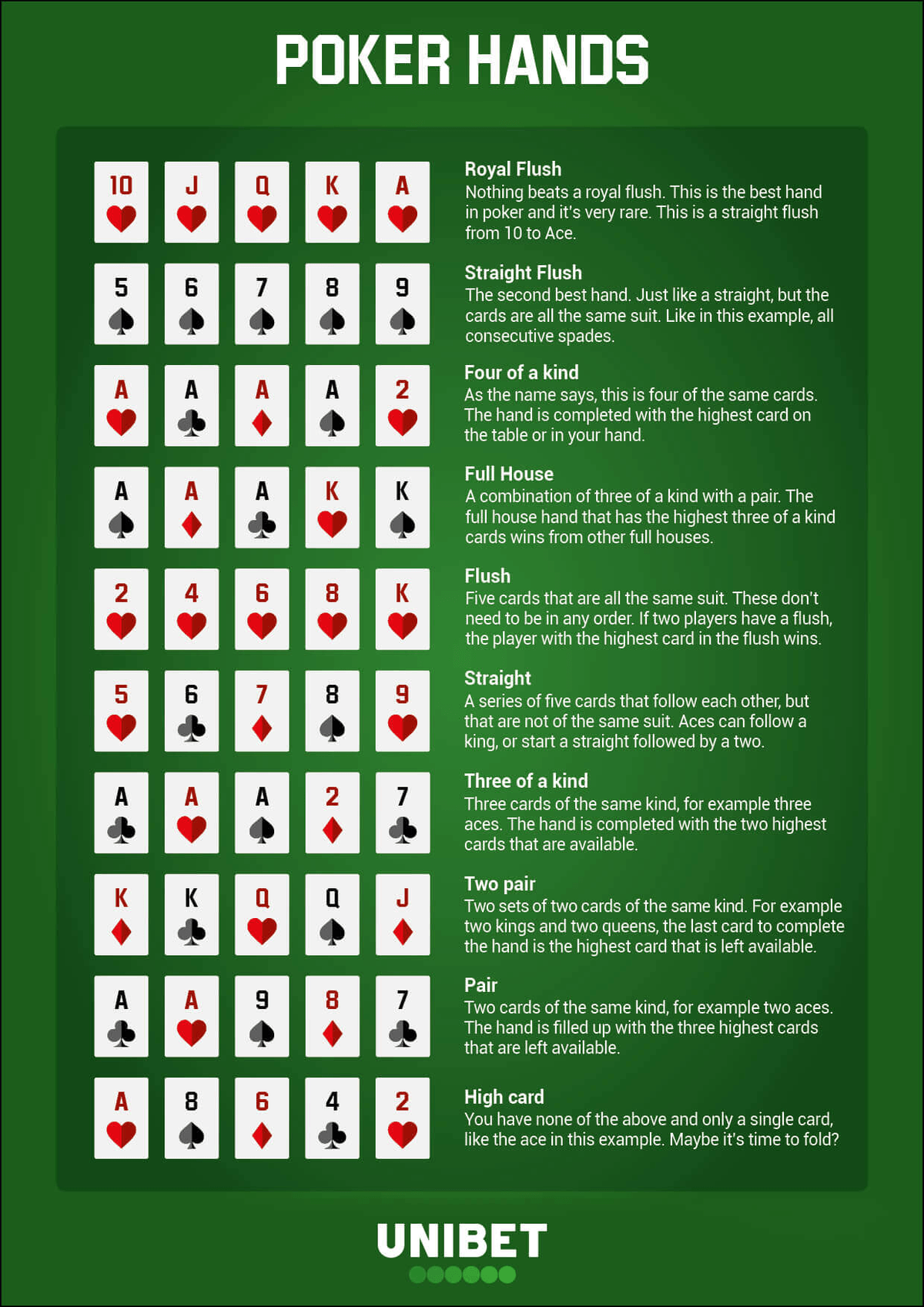
Note that this is not 6 times the hold'em probability since we must specify probabilities for the 3rd and 4th cards before multiplying by 6 in order to exclude another ace. If you want an example of mutually exclusive hands, the AAxx hands with only 2 aces which the OP asked about would be one, so we can compute their probability as: You cannot simply multiply by 6 since the AAAx and AAAA cases contain more than one pair, so they are not mutually exclusive. Therefore, as we are at the Pre-Flop stage, your chance of winning the hand is 87.86 whereas your opponent has just an 11.66 chance.
This is the exactly the same as 4/52 * 3/51 * 6. Note that your method gives exactly the same result as: You need to count these separately like this, where x is a non-ace: This time you are counting the AAAx cases C(3,2) = 3 times, and the AAAA cases C(4,2) = 6 times.
Obviously those hands aren't desirable in Omaha. If you count them then I think it's exactly 6 times as likely as in holdem. Only if you don't count the 3-ace and 4-ace hands that include AA.